CBSE
Class 10 Class 12
Neils Bohr, a Danish physicist, in 1913 proposed model of the atom which rectified the problems left by Rutherford’s Model. He proposed that
Radiate energy as long as they keep on revolving around the nucleus in a fixed orbit.
The circular path around the nucleus is called orbit, energy level or shell. Energy level is represented by letter – K, L, M, N, .... and so on.
Therefore,
1st orbit is denoted by – K
2nd orbit is denoted by – L
3rd orbit is denoted by – M, and so on.
The orbits are denoted by 1, 2, 3, .... and so on
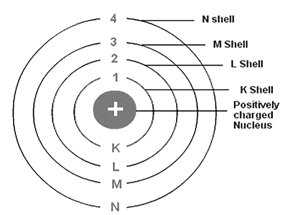
An electron in the hydrogen atom can move around the nucleus in a circular path of fixed radius and energy. These paths are called orbits or energy levels. These orbits are arranged concentrically around the nucleus.
As long as an electron remains in a particular orbit, it does not lose or gain energy and its energy remains constant.
When a transition occurs between two stationary states that differ in energy, the frequency of the radiation absorbed or emitted can be calculated
An electron can move only in those orbits for which its angular momentum is an integral multiple of h/2π
Thus an electron can move only in those orbits for which its angular momentum is an integral multiple of h/2π that is why only certain fixed orbits are allowed.
According to Bohr’s theory for hydrogen atom:
a) The stationary states for electron are numbered n = 1,2,3.......... These integral numbers are known as Principal quantum numbers.
b) The radii of the stationary states are expressed as :
Thus the radius of the first stationary state, called the Bohr orbit, is 52.9 pm
The most important property associated with the electron is the energy of its stationary state. It is given by the expression
where RH is called Rydberg constant and its value is 2.18 × 10–18 J.
Line spectrum observed in case of the hydrogen atom. According to Bohr, radiation is absorbed when the electron moves from the smaller orbit to a higher principal quantum number where the radiation (energy) is emitted.
The distribution of electrons into orbitals of an atom is called its electronic configuration.
The electronic configuration of different atoms can be represented in two ways. For
example :
(i) sa pb dc ...... notation
(ii) Orbital diagram
The electromagnetic spectrum is a continuous spectrum. It consists of a range of electromagnetic radiations arranged in the order of increasing wavelengths or decreasing frequencies. It extends from radio waves to gamma rays.
Spectrum is also classified as emission and line spectrum.
When an electric discharge is passed through gaseous hydrogen, the H2 molecules dissociate and the energetically excited hydrogen atoms produced emit electromagnetic radiation of discrete frequencies.
The hydrogen spectrum consists of several series of lines.
spectral lines are expressed in terms of wavenumber, then the visible lines of the hydrogen spectrum obey the following formula:
where n is an integer equal to or greater than 3 (i.e., n = 3,4,5,....)
The series of lines described by this formula are called the Balmer series.
The Balmer series of lines are the only lines in the hydrogen spectrum which appear in the visible region of the electromagnetic spectrum.
Rydberg noted that all series of lines in the hydrogen spectrum could be described by the following expression
where n1 =1,2........
n2= n1+ 1, n1+ 2......
The value 109,677 cm–1 is called the Rydberg constant for hydrogen. The first five series of lines that correspond to n1 = 1, 2, 3, 4, 5 are known as Lyman, Balmer, Paschen, Bracket and Pfund series.
Planck's Quantum Theory
The radiant energy is emitted or absorbed not continuously but discontinuously in the form of small discrete packets of energy called ‘quantum’. In case of light, the quantum of energy is called a ‘photon’.
The energy of each quantum is directly proportional to the frequency of the radiation,
i.e. E α υ or E= hυ
where h= Planck’s constant = 6.626 x 10-27 Js
Energy is always emitted or absorbed as an integral multiple of this quantum. E=nhυ
Where n=1,2,3,4,
Black body: An ideal body, which emits and absorbs all frequencies, is called a black body. The radiation emitted by such a body is called black body radiation.
Dual behaviour of electromagnetic radiation- The light possesses both particle and wave-like properties, i.e., light has dual behaviour. Whenever radiation interacts with matter, it displays particle-like properties. (Black body radiation and photoelectric effect) Wave-like properties are exhibited when it propagates(interference an diffraction) When a white light is passed through a prism, it splits into a series of coloured bands known as spectrum.
Spectrum is of two types: continuous and line spectrum
Emission spectrum: The spectrum of radiation emitted by a substance that has absorbed energy is called an emission spectrum.
The absorption spectrum is the spectrum obtained when radiation is passed through a sample of material. The sample absorbs radiation of certain wavelengths. The wavelengths which are absorbed are missing and come as dark lines.
Quantum mechanics is a theoretical science that deals with the study of the motions of the microscopic objects that have both observable wavelike and particle-like properties.
Quantum mechanics was developed independently in 1926 by Werner Heisenberg and Erwin Schrödinger.
Quantum mechanical model of the atom is the picture of the structure of the atom, which emerges from the application of the Schrödinger equation to atoms. The following are the important features of the quantum mechanical model of the atom:
Quantum numbers are those numbers which help us to understand that how orbital occupied by an electron.
It helps us to know the following four things:
There are four quantum numbers that help us to describe the location and behavior of an electron. They are:
Principal Quantum Number
The principal quantum number ‘n’ is a positive integer with a value of n = 1,2,3..
It describes the main energy level and size of an orbital i.e. the distance of the orbital from the nucleus. It also identifies the shell. With the increase in the value of ‘n’, the number of allowed orbital increases and are given by ‘n2’ All the orbitals of a given value of ‘n’ constitute a single shell of an atom and are represented by the following letters
n = 1 2 3 4 ............
Shell = K L M N ............
Azimuthal quantum number
The azimuthal quantum number is also known as orbital angular momentum or subsidiary quantum number (l). It defines the three-dimensional shape of the orbital.
For a given value of n, l can have n values ranging from 0 to n – 1, that is, for a given value of n, the possible value of l are: l = 0, 1, 2, ..........(n–1) For example, when n = 1, value of l is only 0. For n = 2, the possible value of l can be 0 and 1.For n = 3, the possible l values are 0, 1 and 2
There are 4 types of orbitals i.e. s, p, d, and f. The value of l ranges from 0 to n-1.
Magnetic Quantum Number
The third is the magnetic quantum number which is denoted by m. This describes the spatial orientation of the orbital. This can be calculated by (2l + 1)
Thus, for l = 0 the permitted value of
mi = 0, [2(0) + 1] =1 = s orbital
mi = 1, [2(1) + 1] =3 = p orbital
mi = 2, [2(2) + 1] =5 = d orbital
mi = 3, [2(3) + 1] =7 = f orbital
The s-orbital has only 1 possible position, p-orbital has 3 possible positions, d-orbital has 5 possible positions and f-orbital has 7 possible positions.
Spin Projection Quantum Number
The fourth is the spin quantum number (ms). It deals with the spin of an electron in a subshell about an axis. The spin quantum number describes the intrinsic electronic spin in which +1/2 denotes the counterclockwise spin of an electron and −1/2 represents the clockwise spin. The maximum number of electrons in an orbital is two and these two electrons should have opposite spins.
The orbital wave function or ψ for an electron in an atom has no physical meaning. The square of the wave function(i.e.,ψ2) at a point gives the probability density of the electron at that point.
In general, it has been found that ns-orbital has (n – 1) nodes, that is, the number of nodes increases with the increase of principal quantum number n.
The shape of the s-orbital is spherical, p-orbital is dumb-bell shaped. The shapes of d and f orbital are very complex and cannot be determined easily. It has been observed that d-orbital are pear-shaped.
The energy of an electron in a hydrogen atom is determined solely by the principal quantum number.
Thus the energy of the orbitals increases as follows :
1s < 2s = 2p < 3s = 3p = 3d <4s = 4p = 4d = 4f <
The orbitals having the same energy are called degenerate.
The filling of electrons into the orbitals of different atoms takes place according to the Aufbau principle which is based on Pauli’s exclusion principle, the Hund’s rule of maximum multiplicity and the relative energies of the orbitals.
Aufbau principle states: In the ground state of the atoms, the orbitals are filled in order of their increasing energies.
In other words, electrons first occupy the lowest energy orbital available to them and enter into higher energy orbitals only after the lower energy orbitals are filled.
The order in which the energies of the orbitals increase and hence the order in which
the orbitals are filled is as follows:
1s, 2s, 2p, 3s, 3p, 4s, 3d, 4p, 5s, 4d, 5p, 4f,5d, 6p, 7s...
Pauli Exclusion Principle: No two electrons in an atom can have the same set of four quantum numbers. Pauli exclusion principle can also be stated as: 'Only two electrons may exist in the same orbital and these electrons must have opposite spin.'
This means that the two electrons can have the same value of three quantum numbers n, l and ml, but must have the opposite spin quantum number.
The maximum number of electrons in the shell with principal quantum number n is equal to 2n2.
Hund's principle: It states that pairing of electron in the orbitals belonging to the same subshell (p,d or f) does not take place until each orbital belonging to that subshell has got one electron each i.e., it is singly occupied.
The exactly half-filled and fully filled orbitals have greater stability than other configurations.
The reason for their stability is symmetry and exchange energy.
(a).Symmetry: The half-filled and fully-filled orbitals are more symmetrical than any other configuration and symmetry leads to greater stability.
(b). Exchange Energy: The electrons present in the different orbitals of the same sub-shell can exchange their positions. Each such exchange leads to the decrease in energy known as exchange Energy. Greater the number of exchanges, greater the exchange energy and hence greater the stability. As the number of exchanges that take place in the half-filled and fully-filled orbitals is maximum, thus exchange energy is maximum and hence maximum stability.
Many attempts were made to develop a more suitable and general model for the atom. Two important developments which contributed significantly to the formulation of such a model were:
The French, physicist, de Broglie in 1924 proposed that matter also exhibit dual behaviour such that particle and wave like properties.
It means that electron has also momentum and wavelength as a photon.
De Broglie, gave a relation between the wavelength () and momentum (p) of the material particle.
where m is the mass of the particle, v its velocity and p its momentum. de Broglie’s the prediction was confirmed experimentally when it was found that an electron beam undergoes diffraction, a phenomenon characteristic of waves.
It states that it is impossible to determine simultaneously, the exact position and exact momentum (or velocity) of an electron.
Mathematically, it can be given as in equation
where Δx is the uncertainty in position and Δpx ( or Δvx) is the uncertainty in momentum (or velocity) of the particle.
The radiations which are associated with electrical and magnetic fields are called electromagnetic radiations. When an electrically charged particle moves under acceleration, alternating electrical and magnetic fields are produced and transmitted. These fields are transmitted in the form of waves. These waves are called electromagnetic waves or electromagnetic radiations.
Electromagnetic waves do not require medium and can move in a vacuum.
The electromagnetic radiation is characterised by the properties, known as frequency (v) and wavelength (λ).
Frequency is defined as the number of waves that pass a given point in one second. The SI unit for frequency is (ν) is hertz (Hz,s-1).
The SI Unit of wavelength is meter (m).
In vaccum, all types of electromagnetic radiations, regardless of wavelength, travel at the same speed, i.e., 3.0 × 108m s–1(2.997925 × 108 ms–1, to be precise). This is
called the speed of light and is given the symbol 'c'. The frequency (ν ), wavelength (λ) and velocity of light (c) are related by the equation.
c = ν λ
Wave number is defined as the number of wavelengths per units length. Its units are recoprocal of wavelength unit, i.e., m-1.