 Multiple Choice Questions
Multiple Choice QuestionsThe straight lines x + y = 0, 5x + y = 4 and x + 5y = 4 form
an isosceles triangle
an equilateral triangle
a scalene triangle
a right angled triangle
If z = x + iy, where x and y are real numbers and i = , then the points (x, y) for which is real, lie on
an ellipse
a circle
a parabola
a straight line
The equation 2x2 + 5xy - 12y2 = 0 represents a
circle
pair of non-perpendicular intersecting straight lines
pair of perpendicular straight lines
hyperbola
The number oflines which pass through the point (2, - 3) and are at a distance 8 from the point (- 1, 2) is
infinite
4
2
0
A line passing through the point of intersection of x + y = 4 and x - y = 2 makes an angle with the x-axis. It intersects the parabola y2 = 4(x - 3) at points (x1, y1) and (x2, y2) respectively. Then, is equal to
The line joinining and , where a b, is produced to the point M(x, y) so that AM : MB = b : a. Then, is equal to
0
1
- 1
a2 + b2
The number of integer values of m, for which the x - coordinate of the point of intersection of the lines 3x + 4y= 9 and y =mx + 1 is also an integer, is
0
2
4
1
If a straight line passes through the point () and the portion of the line intercepted between the axes is divided equally at that point, then is
0
1
2
4
A straight line through the point of intersection of the lines x + 2y = 4 and 2x + y = 4 meets the coordinate axes at A and B. The locus of the mid-point of AB is
3(x + y) = 2xy
2(x + y) = 3xy
2(x + y) = xy
x + y = 3xy
 Short Answer Type
Short Answer TypeFind the image of the point (- 8, 12) with respect to the line 4x + 7y + 13 = 0.
Let image of a point be B (h, k). Mid point of AB is B ![]() which lies on a line
which lies on a line
4x + 7y + 13 = 0
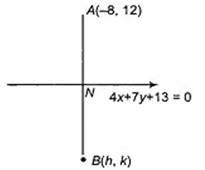
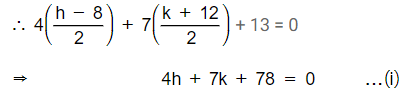
Slope of given line is -4/7, then slope of line AB is 7/4

On solving Eqs. (i) and (ii), we get
k = - 2,h = - 16.
Hence, required image is (- 16, - 2).
