Two 20 g flatworms climb over a very thin wall, 10 cm high. One of the worms is 20 cm long, the other is wider and only 10 cm long. which of the following statement is correct regarding them?

• 20 cm worm has done more work against gravity

• 10 cm worm has done more work against gravity

• Both worms have done equal work against gravity

• Ratio of work done by both the worms is 4:5

A rocket is intended to leave the Earth's gravitational field. The fuel in its main engine is a little less than the amount that is necessary and an auxiliary engine, (only capable of operating for a short time) has to be used as well. When is it best to switch on the auxiliary engine?

• at take-off

• When the rocket has nearly stopped with respect to the Earth

• It doesn't matter

• Can't say

A cylindrical tube of uniform cross-sectional area A is fitted with two air tight frictionless pistons. The pistons are connected to each other by a metallic wire. Initially, the pressure of the gas is p0 and temperature is T0, atmospheric pressure is also p₀. Now, the temperature of the gas is increased to 2T₀, the tension of the wire will be

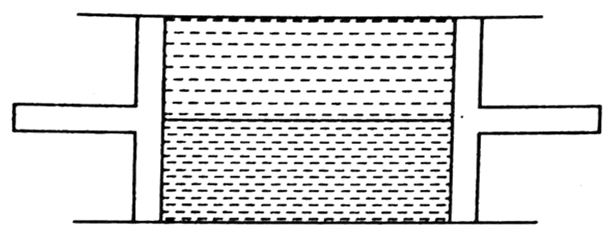
• 2p₀A

• p₀A

• p₀A/2

• 4p₀A

A body is projected vertically upwards. The times corresponding to height h while ascending and while descending are t₁ and t₂, respectively.

• $\frac{\mathrm{g}\sqrt{{\mathrm{t}}_1{\mathrm{t}}_2}}{2}$

• $\frac{\mathrm{g}({\mathrm{t}}_1 +{\mathrm{t}}_2)}{2}$

• $\mathrm{g}\sqrt{{\mathrm{t}}_1{\mathrm{t}}_2}$

• $\mathrm{g}\frac{{\mathrm{t}}_1{\mathrm{t}}_2}{({\mathrm{t}}_1+{\mathrm{t}}_2)}$

The x and y coordinates of a particle moving in a plane are given be x(t) = acos(pt) and y(t) = bsin(pt) where a, b (<a) and p are positive constants of appropriate dimensions and t is time. Then , which of the following is not true?

• The path of the particle is an ellipse

• Velocity and acceleration of the particle are perpendicular to each other at t = π/2p

• Acceleration of the particle is always directed towards a fixed point

• Distance travelled by the particle in the time interval between t = 0 and t = π/2p is a

A skier starts from rest at point A and slides down the hill without turning or breaking. The friction coefficient is μ. When he stops at point B, his horizontal displacement is S. what is the height difference between points A and B?

(The velocity of the skier is small so that the additional pressure on the snow due to the curvature can be neglected. Neglect also the friction of air and the dependence of μ on the velocity of the skier.)

• h = μS

• h = μ/S

• h = 2μS

• h = μS²

A bicycle wheel rolls without slipping on the horizontal floor. Which one of the following is true about the motion of points on the rim of the wheel, relative to the axis at the wheel's centre?

• Points near the top move faster than points near the bottom

• Points near the bottom move faster than points near the top

• All points on the rim move with the same speed

• All points have the velocity vectors that are pointing in the radial direction towards the centre of the wheel

The planets with radii R₁ and R₂ have densities ρ1, ρ2 respectively. Their atmospheric pressure is p1 and p2 respectively. Therefore, the ratio of masses of their atmospheres, neglecting variation of g within the limits of the atmosphere is

• $\frac{{\mathrm{\rho }}_1{\mathrm{R}}_2{\mathrm{\rho }}_1}{{\mathrm{\rho }}_2{\mathrm{R}}_1{\mathrm{\rho }}_2}$

• $\frac{{\mathrm{\rho }}_1{\mathrm{R}}_2{\mathrm{\rho }}_2}{{\mathrm{pP}}_2{\mathrm{R}}_1{\mathrm{\rho }}_1}$

• $\frac{{\mathrm{p}}_1{\mathrm{R}}_1{\mathrm{\rho }}_1}{{\mathrm{p}}_2{\mathrm{R}}_2{\mathrm{\rho }}_2}$

• $\frac{{\mathrm{p}}_1{\mathrm{R}}_1{\mathrm{\rho }}_2}{{\mathrm{p}}_2{\mathrm{R}}_2{\mathrm{\rho }}_1}$

A thin symmertical double convex lens of refractive index μ₂ = 1.5 is placed between a medium of refractive index μ₁ = 1.4 to the left and another medium of refractive index μ₃ = 1.6 to the right. Then, the system behaves as

• a convex lens

• a concave lens

• a glass plate

• a convexo-concave lens

The upper half of an inclined plane of inclination θ is perfectly smooth while the lower half rough. A block starting from rest at the top of the plane will again come to  rest at the bottom if the coefficient of friction between the block and the lower half of the plane is given by

• μ = 2 tanθ

• μ = tanθ

• μ = 2/(tan θ)

• μ = 1/ tan θ